Department of Mathematics
Spring 2010

MATH 418 - Partial Differential Equations

Class Webpage

Class Resources:

books & lecture notes (reserve list)

webct & discussion group (webct.sfu.ca)

note: additional content for math 418 can be found on the webct page

this website will also function as a back-up site in the event of a webct problem

This Week in MATH 418:

08 September: warm-up problems, minor typos corrected from the version handed out in lecture

07 September: writing guide

30 August

first lecture: wed 08 sept, 12:30, AQ5016

best random student quote (facebook) found by accidental google search:

May have to drop Partial Differential Equations -- don't let the "partial" fool you -- this class is full-on TOUGH! Of course, it's been 20 years since I've taken a math class. That may have something to do with it, ya think?!

... (not my PDE student) but while we're wondering, what's so "ordinary" about those DEs anyway?

syllabus: updated office hours

student info form: bring competed form to first lecture

25 June: required text -- partial differential equations (strauss); online additional text -- PDEs in action: from modelling to theory (salsa); online coffee table book for PDEs -- applied PDEs: a visual approach (markowich); online book on PDEs with maple -- PDEs & boundary value problems with Maple V (articolo); online book on computational PDEs -- introduction to PDEs: a computational approach (tveito & winther); online text for review of BVPs -- BVPs & PDEs (powers); online book on advanced PDE analysis -- an introduction to PDEs (renardy & rogers)

25 June: course abstract

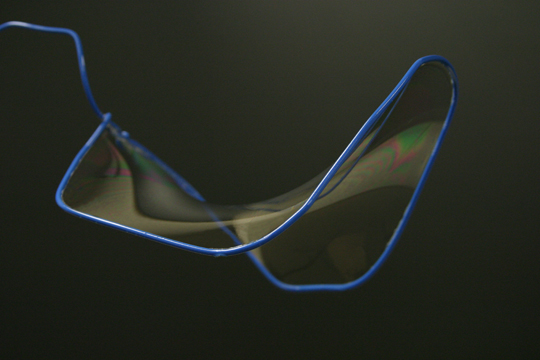
These images are pictorializations of the three basic linear PDEs. The Gaussian blurring of images is a 2D application of diffusion. The slight geometrical distortions of a soap film can be described by the Laplace equation. An acoustic mode of a flat plate is visualized by a Chladni pattern that is related to solutions of the Helmholtz PDE.