STAT 330: 98_1

Assignment 4 Solutions

  1. Chapter 6 Q 10:

    1. Recall the facts: tex2html_wrap_inline52 , tex2html_wrap_inline54 and tex2html_wrap_inline56 Then tex2html_wrap_inline58 which is more than tex2html_wrap_inline60 so that tex2html_wrap_inline62 is not an unbiased estimate of tex2html_wrap_inline60
    2. On the other hand tex2html_wrap_inline66 which is tex2html_wrap_inline60 if k=1/n.

  2. Chapter 6 Q 14:

    1. 525-202+1 = 324.
    2. This estimate will be right if we have seen the plane with the largest number and that with the smallest number. Otherwise our estimate is an underestimate. Thus the average value of our estimator (or its expected value) must be smaller than its largest possible value which is the true number of planes, that is, the parameter value. In other words this estimate (which happens to be the maximum likelihood estimate) is very biased.

  3. Chapter 6 Q 16:

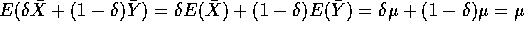    1. tex2html_wrap_inline72 so that this estimate is unbiased for any tex2html_wrap_inline74 .
    2. Var( tex2html_wrap_inline76 Var( tex2html_wrap_inline78 Var( tex2html_wrap_inline80 . The derivative of this with respect to tex2html_wrap_inline74 is tex2html_wrap_inline84 which is 0 when tex2html_wrap_inline86 or

      displaymath88

    3. Chapter 6 Q 22: The first population moment, the mean, is

      displaymath90

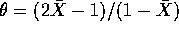      This is equal to tex2html_wrap_inline92 when tex2html_wrap_inline94 or tex2html_wrap_inline96 . In this case tex2html_wrap_inline98 and we get 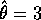tex2html_wrap_inline100 .

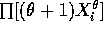    4. The likelihood is tex2html_wrap_inline102 and the log likelihood is

      displaymath104

      The derivative of this with respect to 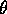tex2html_wrap_inline106 is tex2html_wrap_inline108 which is 0 when tex2html_wrap_inline110 which gives the estimate ?. (I haven't worked it out yet.)

  4. Chapter 6 Q 32:

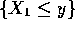    1. The event tex2html_wrap_inline112 is the same as the intersection of the events tex2html_wrap_inline114 , tex2html_wrap_inline116 . Since these events are independent we have, for tex2html_wrap_inline118 ,

      eqnarray24

      The derivative of this with respect to y is the density of Y so the density is tex2html_wrap_inline124 which is part a).

    2. We are asked to calculate the mean of Y which is

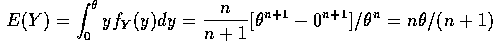
      displaymath128

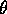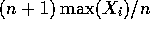      which is not tex2html_wrap_inline106 . Thus Y is biased but tex2html_wrap_inline134 so that tex2html_wrap_inline136 is unbiased.

  5. Chapter 6 Q 34:

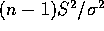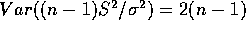    1. When the population distribution is normal we will see that tex2html_wrap_inline138 has a chi-squared distribution with n-1 degrees of freedom, which is a Gamma distribution with shape (n-1)/2 and scale 2. This permits us to prove the hint since tex2html_wrap_inline146 and tex2html_wrap_inline148 . It follows that tex2html_wrap_inline150 has mean tex2html_wrap_inline152 , bias tex2html_wrap_inline154 and variance tex2html_wrap_inline156 . Thus the mean squared error of tex2html_wrap_inline150 is

      displaymath160

      which is a minimum when 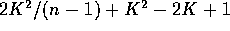tex2html_wrap_inline162 is minimized. Take the derivative with respect to K and set it equal to 0 to get 4K/(n-1)+2K = 2 whose solution is K=(n-1)/(n+1).

  6. Chapter 6 Q 38:

    1. Here we have to multiply together the densities of all the X's and all the Y's. If we take logarithms we get the following log likelihood:

      displaymath174

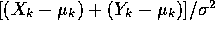      The derivative with respect to tex2html_wrap_inline176 is simply tex2html_wrap_inline178 which is 0 when tex2html_wrap_inline180 . Put this in for each tex2html_wrap_inline176 and note that

      displaymath184

      Now take the derivative with respect to tex2html_wrap_inline186 to get

      displaymath188

      which is 0 when

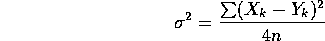
      displaymath190

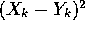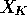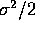    2. The expected value of tex2html_wrap_inline192 is just tex2html_wrap_inline194 because tex2html_wrap_inline196 and tex2html_wrap_inline198 have the same mean. But tex2html_wrap_inline200 so that the expected value of the mle is tex2html_wrap_inline202 . An unbiased estimate is obtained by multiplying by 2 to get

      displaymath204


Richard Lockhart
Fri Feb 6 22:47:52 PST 1998