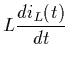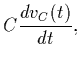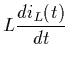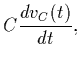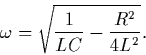Linear RLC circuits are described by differential equations of the second order.

From the KVL:

v_s(t) = v_R(t) + v_C(t) + v_L(t)

and KCL:

i_R(t) = i_C(t) = i_L(t) .

Since

v_R(t) = R i(t)

$$L \frac {d {i_L}(t)}{dt }$$ v_L(t) =

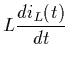$$C \frac {d {v_C}(t)}{dt } ,$$ i_C(t) =

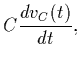the second order differential equation in terms of v_C(t) is:

$${v_s}(t) = LC \frac {d^2 {v_C}(t)}{dt^2 } + RC \frac {d {v_C}(t)}{dt } + {v_C}(t) .$$

Natural frequencies (also called time constants) of this RLC circuit can be found by solving the quadratic equation:

LC s² + RC s + 1 = 0 .

They are of the form:

$$s _{1/2} = - \alpha \pm j \omega , j^2 = -1.$$

where

$$\alpha = \frac{R}{2L} \nonumber$$

and

$$\omega = \sqrt{ \frac{1}{LC} - \frac{R^2}{4L^2}} . \nonumber$$

Note that for a circuit with positive R, L, and C, the real part of the natural frequencies will always be negative.

The response of the circuit depends one the relative values of R, L, and C. We distinguish cases:

• Over-damped circuit: s 1 and s 2 real and distinct
• Under-damped circuit: s 1 and s 2 complex conjugate numbers, and
• Critically damped circuit: s 1 = s 2 .