2. Fluid-Structure Interaction and the Immersed Boundary Method
Applying the immersed boundary method to simulate the interaction of an
elastic, deformable interface with an incompressible fluid
flow. My approach to studying complex fluid-structure interaction
problems is two-fold:
- Using analytical techniques to investigate the stability and
dynamics of solutions to the immersed boundary equations.
- Applying these analytical insights to better understand the
behaviour of numerical schemes and to help design new and more
efficient algorithms.
In addition to this theoretical work, I am particularly interested in
using the immersed boundary or IB method to simulate various problems
motivated by applications in engineering and biology. My current
projects include:
-
Analytical studies of immersed boundaries: I have applied
techniques from linear stability analysis and asymptotics to
study the stability of solutions to the IB
equations. This information is extremely useful since no
exact solutions are known, and it also provides insight into the
behaviour of commonly used numerical approaches. This work formed
the bulk of my PhD thesis
and was also published in a number of journal articles
[J6],
[J2].
I have also investigated the phenomenon of parametric resonance
in the context of parametrically-forced immersed boundaries
[J11],
such as one might see in the
heart [J32] or inner ear
[J28].
-
Particle suspensions: I have used the immersed
boundary method to simulate the rotational dynamics of a single
flexible fibre suspended within a planar shear flow. Extensive
computations in 2D and 3D
[J5],
[C5],
[J27],
show close correspondence with both experimental results and
(approximate) analytical solutions. I have also studied
sedimentation of rigid circular particles in 2D [J26]. My interest
in these problems is partially motivated by the study wood pulp
fibres in the papermaking industry.
| 2D single-fiber orbits |
|
3D semi-dilute suspensions |
 |
|
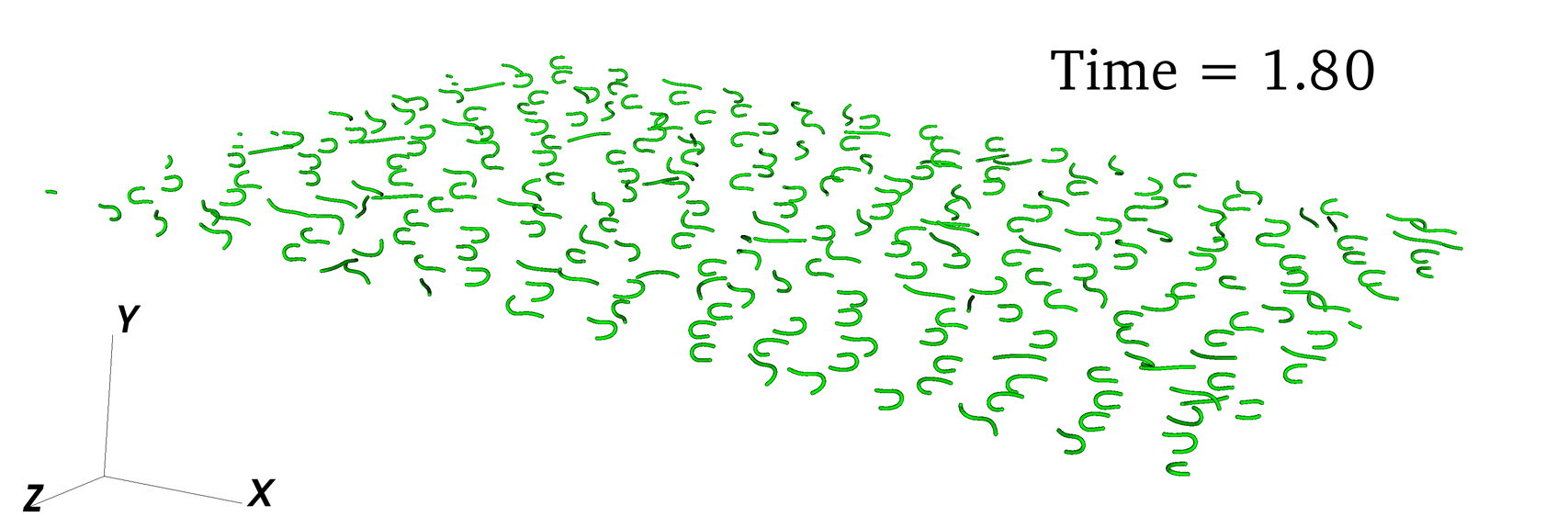 |
-
Membrane transport in porous vesicles: Vesicles are
fluid-filled membranes that transport nutrients and other substances
within cells through a selectively permeable membrane. To deal with
the case where vesicles undergo large deformations and changes in
volume, I have extended the IB method to handle porous immersed
boundaries and developed a cute approximate analytical solution to
go along with it
[J14].
-
Biofilm growth and deformation.
-
Swimming dynamics of marine organisms: The IB method has had
a long history of simulating the swimming motions of marine worms,
flagellated cells, spirochetes, leeches, and other similar
organisms. I have a particular interest in the study of squid and
jellyfish, whose muscle has a fiber architecture that lends itself
ideally to an immersed boundary description. This is ongoing work
. . .
| 2D IB simulation (with MatIB) |
|
Chrysaora fuscescens |
 |
|
 |
I have collected a few older images and animations from these projects here.
|
[ Home ]
|
Last modified: Tue Apr 21 2020
|







